Question

In the United States, 36 percent of the people have a blood type that is A positive. From a random sample of 150 people from Norway, 66 had a blood type that was A positive. Consider a hypothesis test to investigate whether the proportion of people in Norway with a blood type of A positive is different from that in the United States.

Determine the standard deviation used to calculate the test statistic for the one-sample z-test.

Answer 1

The standard deviation used in the test statistic is 0.04.

Explanation:

A single proportion z-test can be used to determine whether the proportion of people in Norway with a blood type of A positive is different from that in the United States.

The proportion of people in Norway with a blood type of A positive is 36% or 0.36.

The hypothesis can be defined as:

H₀: The proportion of people in Norway with a blood type of A positive is 36%, i.e. p = 0.36.

Hₐ: The proportion of people in Norway with a blood type of A positive is different from 36%, i.e. p ≠ 0.36.

The test statistic is:

`z=\frac{\hat p-p}{\sqrt{(p(1-p))/(n)}}`

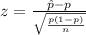
The information provided is:

p = 0.36

n = 150

Compute the standard deviation used in the test statistic as follows:

`\sigma=\sqrt{(p(1-p))/(n)}`

`=\sqrt{(0.36* (1-0.36))/(150)}`

`=√(0.001536)\\=0.03919184\\\approx 0.04`

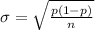
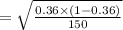
Thus, the standard deviation used in the test statistic is 0.04.