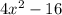Answer:
We need to add the expression:
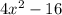
Explanation:
As the first step, add the first two polynomials listed in order to find what their sum is. Do such by combining like terms:
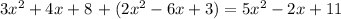
now, you need to find another polynomial (let's call it P(x)) such that added to the one we just found above, will render
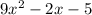 as a result.
as a result.
Putting this phrase in mathematical terms, we write:
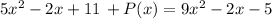
Now we solve for this unknown P(x) in this equation, by subtracting each of the terms that are accompanying it on the left side of the equation. We do one at a time for clarity:
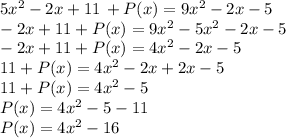
Therefore, the expression we need to add is: