Question

Brad is putting up a 12 foot diameter circular flower garden in his yard. He will put

plastic edging along the flower bed. How many feet of edging will Brad need to
enclose his flower garden? LEAVE IN TERMS OF PLAND INCLUDE A SHORT
DESCRIPTION OF HOW YOU ARRIVED AT YOUR ANSWER.

​

Answer 1

Brad needs 37.68 ft of plastic edging to enclose the flower garden, in terms of "pi" it is 12π only.

Explanation:

Given:

Diameter of the circular flower garden = 12 ft

We have to find the measure of the plastic edging Brad need to have.

So,

We have to find the length of the edge of the circular garden for circular object the measure of its length has a special name we call it circumference.

And it is very well known to us that:

For any circle the ratio of its circumference and its diameter is equivalent to pie (π) which is equal to 3.14

Then,

⇒
`(Circumference)/(Diameter) = \pi`

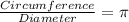
In the above question we have to find the measure of its circumference.

⇒
`Circumference= \pi D`

⇒
`Circumference= \pi (12)`

⇒
`Circumference= 12\pi` ft

⇒ Or

⇒
`Circumference= 12(3.14)`

⇒
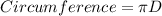
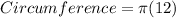
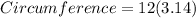
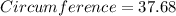`Circumference= 37.68` ft

Brad needs 37.68 ft of plastic edging to enclose the flower garden, in terms of "pi" it is 12π only.