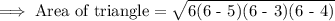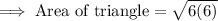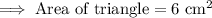Question

Find the area.
5cm
3cm
4 cm

Answer 1

6 cm²

Explanation:

Since 3 side lengths are given, I assume that this figure is a triangle. The area of a triangle can be determined by using hero's formula.

`\text{Area of triangle} = \sqrt{\text{s(s - a)(s - b)(s - c)}`

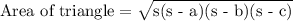
⇒ s = Perimeter/2

⇒ a, b, and c = side lenths of triangle

Replacing a, b, and c as the given side lengths:

`\implies \text{Area of triangle} = \sqrt{\text{s(s - 5)(s - 3)(s - 4)}`

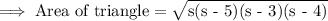
Determining the perimeter of the triangle:

`\text{Perimeter of triangle = Sum of it's side lengths}`

`\implies \text{Perimeter of triangle =}\ 5 + 4 + 3`

`\implies \text{Perimeter of triangle =}\ 12 \ \text{cm}`

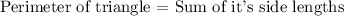
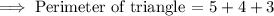
Determining the half of perimeter:

`\text{Half of perimeter} = s = \frac{\text{Perimeter}}{2}`

`\implies s = (12)/(2)`

`\implies s = 6`

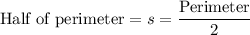
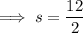
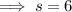
Replacing the value of "s" in the formula:

`\implies \text{Area of triangle} = \sqrt{\text{6(6 - 5)(6 - 3)(6 - 4)}`

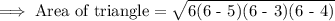
Evaluating the area:

`\implies \text{Area of triangle} = \sqrt{\text{6(6 - 5)(6 - 3)(6 - 4)}`

`\implies \text{Area of triangle} = \sqrt{\text{6(1)(3)(2)}`

`\implies \text{Area of triangle} = \sqrt{\text{6(6)}`

`\implies \text{Area of triangle} = 6 \ \text{cm}^(2)`