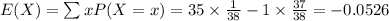Question

A Nevada roulette wheel had 38 pockets, labeled as 0 00 1 2 3 ... 35 36. One bet is single number. If you bet $1 on a number (any one between 1 and 36) and the number comes up, you get the dollar back together with $35 in winnings; otherwise, you lose your dollar.

A.) Find the probability model of your net gain.

B.) Find the mean of your net gain for one bet.

Answer 1

-0.0526

Explanation:

Let X be the random variable denoting the net gain(in dollars) for a single trial(one bet).

Assuming that each number in the wheel is equally likely, probability of the outcome being a victory is
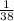`(1)/(38)` and probability of failure is
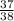`(37)/(38)`. For a win, X takes value 35 and for a loss X takes value -1. So the model is,

`P(X=35) = (1)/(38)`

`P(X=-1) = (37)/(38)`

`P(X=i) = 0 \;if\; i \\eq 38, i\\eq -1`

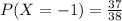
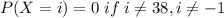
The mean for one bet is
`E(X) = \sum xP(X=x) = 35*(1)/(38) - 1*(37)/(38) = -0.0526`