Question

The lifetime of certain type of light bulb is normally distributed with a mean of 1000 hours and a standard deviation of 110 hours. A hardware store manager claims that the new light bulb model has a longer average lifetime. A sample of 10 from the new light bulb model is obtained for a test. Consider a rejection region Find the probability of a type I error (round off to third decimal place).

Answer 1

The probability of a type I error is 0.500.

Explanation:

A null hypothesis is a hypothesis of no difference. It is symbolized by H₀.

A Type-I-error is the rejection of an H₀ when indeed the H₀ is true. It is symbolized by α.

The hypothesis to test whether the store manager's claim, that the new light bulb model has a longer average lifetime, or not can be defined as:

H₀: The average lifetime of the new light bulb model is 1000 hours, i.e. μ = 1000.

Hₐ: The average lifetime of the new light bulb model is more than 1000 hours, i.e. μ > 1000.

Now, the type I error will be committed if we conclude that the mean lifetime of new bulbs is more than 1000 hours when in fact it is not.

The rejection region is defined as:

`P(\bar X>1000)`

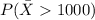
Given:

σ = 110 hours

n = 10

Compute the value of
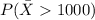`P(\bar X>1000)` as follows:

`P(\bar X>1000)=P((\bar X-\mu)/(\sigma/√(n))>(1000-1000)/(110/√(10)))`

`=P(Z>0)\\=1-P(Z<0)\\=1-0.50\\=0.50`

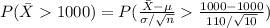
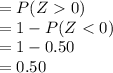
*Use a z-table for the probability.

The probability that the mean lifetime of new bulbs is more than 1000 hours is 0.50.

Hence, the probability of a type I error is 0.500.