Question

Mr. Cotton has to give a lecture at 3:30. From past experience, we know that Mr. Cotton will arrive from 1 minute early up to 5 minutes late and is equally likely to arrive at any of these times (So it’s uniformly distributed). He will begin the lecture 2 minutes after he arrives.

(a) What is the probability that Mr. Cotton begins the lecture after 3:35?
(b) What is the expected time that the lecture will begin?

Answer 1

a) 2/7

b) 3:33

Explanation:

a) We are told from experience Mr. cotton arrives the class from 1 minute early (3;30-1minute) = 3:29 and he starts the class after 2 minutes (3;29 + 2mins) = 3: 31

We are also told he arrives up to 5 minutes late.

Therefore,

•If he arrives at 3:30, he will start at 3:32

•If he arrives at 3:31, he will start at 3:33

•If he arrives at 3:32, he will start at 3:34

•If he arrives at 3:33, he will start at 3:35

•If he arrives at 3:34, he will start at 3:36

•If he arrives at 3:35, he will start at 3:37

Therefore, to find the probability that he starts after 3:35, we have:

•Total number of outcomes = 7

•Number of times class starts after 3:35 = 2

Thus, the probability wil be = 2/7

b) The expected time that the lecture will begin =
`(-1+0+1+2+3+4+5+6+7)/(7)`

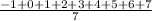
= 3.85 minutes late =

Lecture begins at 3:33