Question

The average maximum monthly temperature in Campinas, Brazil is 29.9 degrees Celsius. The standard deviation in maximum monthly temperature is 2.31 degrees. Assume that maximum monthly temperatures in Campinas are normally distributed. What percentage of months would have a maximum temperature of 32 degrees or higher? Round your answer to one decimal place.

Answer 1

`P(X>32)=P((X-\mu)/(\sigma)>(32-\mu)/(\sigma))=P(Z>(32-29.9)/(2.31))=P(z>0.909)`

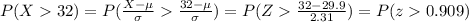
And we can find this probability with the complement rule and using the normal standard distributon table or excel we got:

`P(z>0.909)=1-P(z<0.909)=1-0.818=0.182`

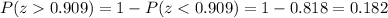
And if we convert this to a % we got 18.2 % of maximum temperatures higher or equal than 32 C

Explanation:

Previous concepts

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

The Z-score is "a numerical measurement used in statistics of a value's relationship to the mean (average) of a group of values, measured in terms of standard deviations from the mean".

Solution to the problem

Let X the random variable that represent the maximum monthly temperature of a population, and for this case we know the distribution for X is given by:

`X \sim N(29.9,2.31)`

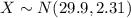
Where
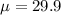`\mu=29.9` and
`\sigma=2.31`

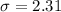
We are interested on this probability

`P(X>32)`

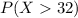
And the best way to solve this problem is using the normal standard distribution and the z score given by:

`z=(x-\mu)/(\sigma)`

If we apply this formula to our probability we got this:

`P(X>32)=P((X-\mu)/(\sigma)>(32-\mu)/(\sigma))=P(Z>(32-29.9)/(2.31))=P(z>0.909)`

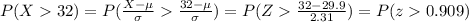
And we can find this probability with the complement rule and using the normal standard distributon table or excel we got:

`P(z>0.909)=1-P(z<0.909)=1-0.818=0.182`

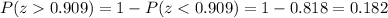
And if we convert this to a % we got 18.2 % of maximum temperatures higher or equal than 32 C