Question

Which expressions are equivalent??

Answer 1

Given:

The given expression is
`2\left((3)/(4) x+7\right)-3\left((1)/(2) x-5\right)`

We need to determine the equivalent expression.

Option A: -1

Solving the expression, we get;

`(3)/(2) x+14-(3)/(2) x+15`

Simplifying, we get;

`14+15=29`

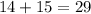
Thus, the expression
`2\left((3)/(4) x+7\right)-3\left((1)/(2) x-5\right)` is not equivalent to -1.

Hence, Option A is not the correct answer.

Option B: 29

Solving the expression, we get;

`(3)/(2) x+14-(3)/(2) x+15`

Simplifying, we get;

`14+15=29`

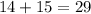
Thus, the expression
`2\left((3)/(4) x+7\right)-3\left((1)/(2) x-5\right)` is equivalent to 29.

Hence, Option B is the correct answer.

Option C:
`2\left((3)/(4) x+7\right)+(-3)\left[(1)/(2) x+(-5)\right]`

Let us rewrite the given expression.

`2\left((3)/(4) x+7\right)+(-3)\left[(1)/(2) x+(-5)\right]`

Thus, the expression
`2\left((3)/(4) x+7\right)-3\left((1)/(2) x-5\right)` is equivalent to
`2\left((3)/(4) x+7\right)+(-3)\left[(1)/(2) x+(-5)\right]`

Thus, Option C is the correct answer.

Option D:
`2\left((3)/(4) x\right)+2(7)+3\left((1)/(2) x\right)+3(-5)`

Rewriting the expression, we get;

`2\left((3)/(4) x+7\right)+(-3)\left[(1)/(2) x+(-5)\right]`

Hence, the expression
`2\left((3)/(4) x+7\right)-3\left((1)/(2) x-5\right)` is equivalent not to
`2\left((3)/(4) x\right)+2(7)+3\left((1)/(2) x\right)+3(-5)`

Thus, Option D is not the correct answer.

Option E:
`2\left((3)/(4) x\right)+2(7)+(-3)\left((1)/(2) x\right)+(-3)(-5)`

Multiplying the terms within the bracket, we get;

`2\left((3)/(4) x\right)+2(7)+(-3)\left((1)/(2) x\right)+(-3)(-5)`

Hence, the expression
`2\left((3)/(4) x+7\right)-3\left((1)/(2) x-5\right)` is equivalent to
`2\left((3)/(4) x\right)+2(7)+(-3)\left((1)/(2) x\right)+(-3)(-5)`

Thus, Option E is the correct answer.