Question

Tanya kicks a ball into the air. The function that models this scenario f(t)= -16t²+96t, where h is the height of the ball in feet and t is the time in seconds. When will the ball hit the ground?

Answer 1

The ball hit the ground at t=6 seconds

Explanation:

we have

`f(t)=-16t^2+96t`

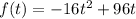
where

f(t) is he height of the ball in feet

t is the time in seconds

we know that

When the ball hit the ground, the height of the ball is equal to zero

so

For f(t)=0

`-16t^2+96t=0`

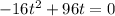
solve for t

Factor the leading coefficient -16t

`-16t(t-6)=0`

the solutions are

t=0, t=6

Remember that

For t=0 ----> f(t)=0 is the initial height of the ball

Fort=6 sec ----> f(t)=0

therefore

The ball hit the ground at t=6 seconds