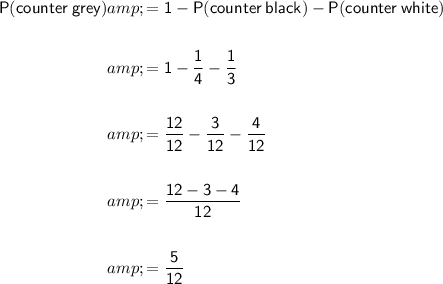
Answer:
1/12
Explanation:
Needed information
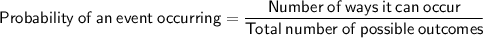
The sum of the probabilities of all outcomes must equal 1
Solution
We are told that the probability that the counter is not black is 3/4.
As the sum of the probabilities of all outcomes must equal 1, we can work out the probability that the counter is black by subtracting 3/4 from 1:


We are told that the probability that the counter is not white is 2/3.
As the sum of the probabilities of all outcomes must equal 1, we can work out the probability that the counter is white by subtracting 2/3 from 1:


We are told that there are black, white and grey counters in the bag. We also know that the sum of the probabilities of all outcomes must equal 1. Therefore, we can work out the probability the counter is grey by subtracting the probability the counter is black and the probability the counter is white from 1: