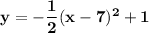Question

Part 3: Use the information provided to write the vertex formula of each parabola. Please show the work

1. Vertex: (-4, -3), Directrix: y = -25/8


2. Vertex: (-8, -7), Directrix: y= -25/4


3. Focus: (7, 1/2), Directrix: y = 3/2

Answer 1

Answer: 1. y = 2(x + 4)² - 3

`\bold{2.\quad y=-(1)/(3)(x+8)^2-7}`

`\bold{3.\quad y=-(1)/(2)(x-7)^2+1}`

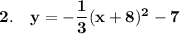
Explanation:

Notes: The vertex form of a parabola is y = a(x - h)² + k

• (h, k) is the vertex
• p is the distance from the vertex to the focus

`\bullet\quad a=(1)/(4p)`

1)

`\text{Vertex}=(-4,-3)\qquad \text{Directrix}:y=-(25)/(8)\\\\\text{Given}:(h, k)=(-4, 3)\\\\p=(-24)/(8)-(-25)/(8)=(1)/(8)\\\\\\a=(1)/(4p)=(1)/(4((1)/(8)))=(1)/((1)/(2))=2`

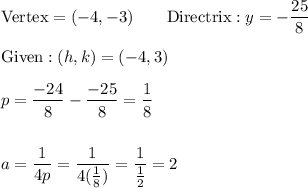
Now input a = 2 and (h, k) = (-4, -3) into the equation y = a(x - h)² + k

y = 2(x + 4)² - 3

******************************************************************************************

2)

`\text{Vertex}=(-8,-7)\qquad \text{Directrix}:y=-(-25)/(4)\\\\\text{Given}:(h, k)=(-8, -7)\\\\p=(-28)/(4)-(-25)/(4)=(-3)/(4)}\\\\\\a=(1)/(4p)=(1)/(4((-3)/(4)))=(1)/(-3)=-(1)/(3)`

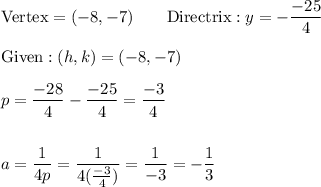
Now input a = -1/3 and (h, k) = (-8, -7) into the equation y = a(x - h)² + k

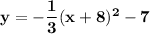
`\bold{y=-(1)/(3)(x+8)^2-7}`

******************************************************************************************

3)

`\text{Focus}=\bigg(7,(1)/(2)\bigg)\qquad \text{Directrix}:y=(3)/(2)`

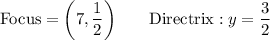
The midpoint of the focus and directrix is the y-coordinate of the vertex:

`(focus+directrix)/(2)=((1)/(2)+(3)/(2))/(2)=((4)/(2))/(2)=(2)/(2)=1`

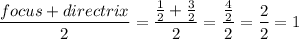
The x-coordinate of the vertex is given in the focus as 7

(h, k) = (7, 1)

Now let's find the a-value:

`p=(2)/(2)-(3)/(2)=(-1)/(2)}\\\\\\a=(1)/(4p)=(1)/(4((-1)/(2)))=(1)/(-2)=-(1)/(2)`

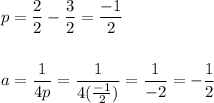
Now input a = -1/2 and (h, k) = (7, 1) into the equation y = a(x - h)² + k

`\bold{y=-(1)/(2)(x-7)^2+1}`