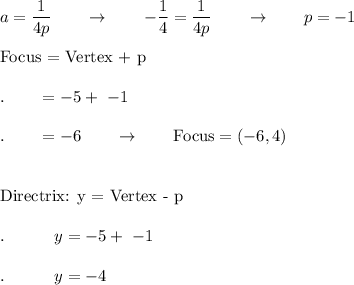Question

Part 2: Identify the vertex, focus, and directrix of each. Then sketch the graph.

1. y = (x + 3)^2 - 6

2. x = -1/4(y - 4)^2 - 5

Answer 1

Answer:
`\bold{1.\quad \text{Vertex}}:(-3,-6)\qquad \text{Focus}:\bigg(-3,-(17)/(4)\bigg)\qquad \text{Directrix}:y=-(19)/(4)`

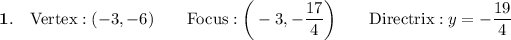
2. Vertex: (-5, 4) Focus: (-6, 4) Directrix: x = -4

Explanation:

The vertex form of a parabola is y = a(x - h)² + k or x = a(y - k)² + h

• (h, k) is the vertex
• p is the distance from the vertex to the focus
• -p is the distance from the vertex to the directrix

`\bullet \quad a=(1)/(4p)`

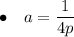
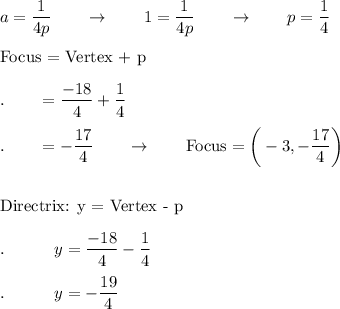
1) y = (x + 3)² - 6 → a = 1 (h, k) = (-3, -6)

`a=(1)/(4p)\qquad \rightarrow \qquad 1=(1)/(4p) \qquad \rightarrow \qquad p=(1)/(4)\\\\\text{Focus = Vertex + p}\\\\.\qquad =(-18)/(4)+(1)/(4)\\\\.\qquad = -(17)/(4)\qquad \rightarrow \qquad \text{Focus}=\bigg(-3,-(17)/(4)\bigg)\\\\\\\text{Directrix: y = Vertex - p}\\\\.\qquad \quad y=(-18)/(4)-(1)/(4)\\\\.\qquad \quad y= -(19)/(4)`

****************************************************************************************

`2.\quad x=-(1)/(4)(y-4)^2-5\qquad \rightarrow \quad a=(1)/(4)\qquad (h, k)=(-5,4)`

`a=(1)/(4p)\qquad \rightarrow \qquad -(1)/(4)=(1)/(4p) \qquad \rightarrow \qquad p=-1\\\\\text{Focus = Vertex + p}\\\\.\qquad =-5+\ -1\\\\.\qquad = -6\qquad \rightarrow \qquad \text{Focus}=(-6,4)\\\\\\\text{Directrix: y = Vertex - p}\\\\.\qquad \quad y=-5+\ -1\\\\.\qquad \quad y= -4`