Question

An airplane flew with the wind for 2.5 hours and returned the same distance against the wind in 3.5 hours. If the cruising speed of the plane was a constant 360 mph in air, how fast was the wind blowing

Answer 1

60 miles per hour.

Explanation:

Let r represent speed of wind blowing in miles per hour.

We have been given that the cruising speed of the plane was a constant 360 mph in air. The speed of the plane is the direction of wind would be
`360+r`.

The speed of the plane is the opposite direction of wind would be
`360-r`.

`\text{Distance}=\text{Speed}* \text{Time}`

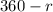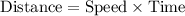
Distance covered in the direction of wind would be
`2.5(360+r)`.

Distance covered in the opposite direction of wind would be
`3.5(360-r)`.

Since both distances are same, so we will get:

`2.5(360+r)=3.5(360-r)`

`900+2.5r=1260-3.5r`

`900-900+2.5r+3.5r=1260-900-3.5r+3.5r`

`6r=360`

`(6r)/(6)=(360)/(6)`

`r=60`

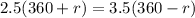
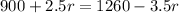
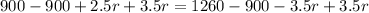
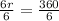
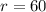
Therefore, the wind is blowing at a rate of 60 miles per hour.