Question

Let’s begin with a simple circular-motion problem in which tension provides the necessary radial acceleration. Suppose you fly a propeller-driven model airplane on a 5.00 m string in a horizontal circle. The airplane, which has a mass of 0.500 kg, flies level and at constant speed and makes one revolution every 4.00 s. How hard must you pull on the string to keep the plane flying in a circle? slader

Answer 1

The force acting on the spring is 6.162 N.

Step-by-step explanation:

Given that,

Mass of the airplane, m = 0.5 kg

The airplane fly on a 5 m string in a horizontal circle.

It flies level and at constant speed and makes one revolution every 4.00 s. We need to find the force with which the force in the string to keep the plane flying in a circle. It is given by :

`F=mr\omega^2`

`\omega=0.25\ rev/s\\\\\omega=1.57\ rad/s`

`F=0.5* 5* (1.57)^2\\\\F=6.162\ N`

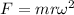
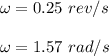
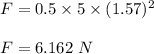
So, the force acting on the spring is 6.162 N.