Question

An octagonal swimming pool has a base area of 22 square feet The pool is 3 feet deep How many cubic feet of water can the pool hold?

Answer 1

The pool can hold 65.84 ft³ of water

Step-by-step explanation:

Given:

Shape of pool = octagonal

Base area of the pool = 22 ft²

Depth of the pool = 3 feet

Volume, V = ?

We know:

Area of octagon = 2 ( 1 + √2) a²

22 ft² = 2 ( 1 + √2 ) a²

`(11)/(1+√(2) ) = a^2`

a² =
`(11)/(2.42)`

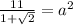
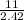
a² = 4.55

a = 2.132 ft

Side length of the octagon is 2.132 ft

We know:

Volume of octagon =
`2(1+√(2) ) X (a)^2 X h`

`V = 2(1+√(2))X (2.132)^2 X 3\\ \\V = 2 ( 2.414) X 4.5454 X 3\\\\V = 65.84 ft^3`

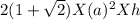
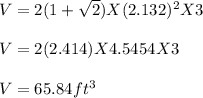
Therefore, the pool can hold 65.84 ft³ of water