Question

The weights of certain machine components are normally distributed with a mean of 8.01 g and a standard deviation of 0.06 g. Find the two weights that separate the top 3% and the bottom 3%. These weights could serve as limits used to identify which components should be rejected. Round to the nearest hundredth of a gram. 8.00 g and 8.02 g 7.88 g and 8.17 g 7.98 g and 8.04 g 7.90 g and 8.12 g

Answer 1

Option D) 7.90 g and 8.12 g

Explanation:

We are given the following information in the question:

Mean, μ = 8.01 g

Standard Deviation, σ = 0.06 g

We are given that the distribution of weights is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

We have to find the value of x such that the probability is 0.03

P(X > x)

`P( X > x) = P( z > \displaystyle(x - 8.01)/(0.06))=0.03`

`= 1 -P( z \leq \displaystyle(x - 8.01)/(0.06))=0.03`

`=P( z \leq \displaystyle(x - 8.01)/(0.06))=0.97`

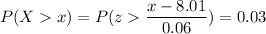
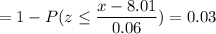
Calculation the value from standard normal z table, we have,

`\displaystyle(x - 8.01)/(0.06) = 1.881\\\\x = 8.12`

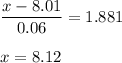
Thus, 8.17 g separates the top 3% of the weights.

P(X < x)

`P( X < x) = P( z < \displaystyle(x - 8.01)/(0.06))=0.03`

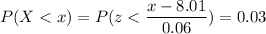
Calculation the value from standard normal z table, we have,

`\displaystyle(x - 8.01)/(0.06) = -1.881\\\\x = 7.90`

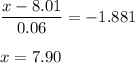
Thus, 7.90 separates the bottom 3% of the weights.

Thus, the correct answer is

Option D) 7.90 g and 8.12 g