Question

A submerged scuba diver looks up toward the calm surface of a freshwater lake and notes that the Sun appears to be 33 ∘ from the vertical. The diver's friend is standing on the shore of the lake.

At what angle above the horizon does the friend see the sun?

Answer 1

The concept necessary to solve this problem is related to Snell's law, which describes the relationship between the angles of incidence of light at the rate of diffraction coefficient of materials, mathematically that is,

`(sin\theta_2)/(sin\theta1) = (n_1)/(n_2)`

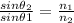
The refractive index for the water is 1.33 and for the air is 1, then replacing we will have,

`n_1 sin\theta_1 = n_2 sin\theta_2`

`(1.00)sin\theta_1 = (1.33)sin(33)`

`\theta_1 = sin^(-1) ((1.33)sin(33))`

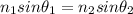
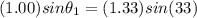
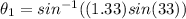
The relation between the angle of incidence and the angle above the horizon at which the friend sees the sun is,

`\theta' = 90\°-\theta_1`

`\theta' = 90\° - sin^(-1) ((1.33)sin(33))`

`\theta' = 43.58\°`

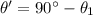
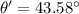
The angle of incidence is greater than the angle of refraction. This is because the incident and refracted angles are inversely proportional to the refractive index of the medium.