Question

A bicyclist of mass 60kg supplies 340W of power while riding into a 5 m/s head wind. The frontal area of the cyclist and bicycle together is 3.7ft2(0.344 m2), the drag coefficient is 0.88, and the coefficient of rolling resistance is 0.007. Determine the speed of the cyclist.Express your answer in mph. (Hint: Lecture Not

Answer 1

87.1 mph

Step-by-step explanation:

We are given that

Mass,m=60 kg

Power,P=340 W

Speed,v=5 m/s

Area,
`A=0.344 m^2`

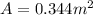
Drag coefficient,
`C_d=0.88`

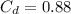
Coefficient of rolling resistance,
`\mu_r=0.007`

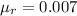
Friction force,
`f=\mu_rmg=0.007* 60* 9.8=4.1 N`

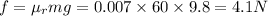
Where
`g=9.8 m/s^2`

Let speed of cyclist=v'

Drag force,
`F_d=(1)/(2)\rho_(air)AC_dv^2`

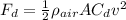
Density of air,
`\rho_(air)=1.225 kg/m^3`

`F_d=(1)/(2)* 1.225* 0.344* 0.88(5)^2=4.635N`

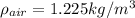
Power,P=
`(F_d+f)* v'`

`340=(4.1+4.635) v'=8.735v'`

`v'=(340)/(8.735)=38.9m/s`

`v'=87.1 mph`

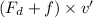
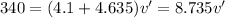
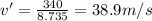
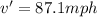
1 m=0.00062137 miles

1 hour=3600 s