Answer:
a.
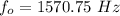
b.
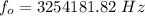 which won't be audible to the humans as the audible capacity of the human ear is from 20 Hz to 20,000 Hz.
which won't be audible to the humans as the audible capacity of the human ear is from 20 Hz to 20,000 Hz.
Step-by-step explanation:
Given:
- speed of jet,
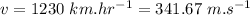
- speed of sound in air,
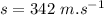
- frequency of sound,
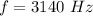
a.
Since the sound source is moving therefore we can determine the observed frequency by the Doppler's effect given as:
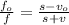
where:
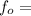 observed frequency when the jet plane approaches the observers
observed frequency when the jet plane approaches the observers
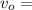 observer's velocity = 0
observer's velocity = 0
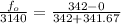
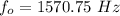
b.
When the plane flies away from the observers then we take its velocity as negative.
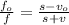
where:
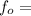 observed frequency when the jet plane flies away from the observers
observed frequency when the jet plane flies away from the observers
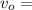 observer's velocity = 0
observer's velocity = 0
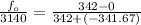
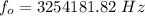 which won't be audible to the humans as the audible capacity of the human ear is from 20 Hz to 20,000 Hz.
which won't be audible to the humans as the audible capacity of the human ear is from 20 Hz to 20,000 Hz.