Given:
Principal= $3000
Rate of interest = 5%
Time = 4 years
n = 1 (compounded annually)
To find:
The balance in the account after 4 years
Solution:
Compound interest formula:
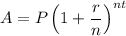
Substitute the given values.
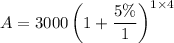
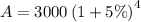
To convert percentage into fraction divide by 100.
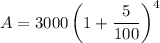
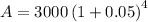
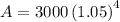
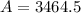
The balance in the account after 4 years is $3464.5.