Question

The Captain has probability \dfrac{1}{2} 2 1 ​ start fraction, 1, divided by, 2, end fraction of hitting the pirate ship. The pirate only has one good eye, so he hits the Captain's ship with probability \dfrac{1}{6} 6 1 ​ start fraction, 1, divided by, 6, end fraction. If both fire their cannons at the same time, what is the probability that both the pirate and the Captain hit each other's ships?

Answer 1

0.083 is the required probability.

Explanation:

We are given the following in the question:

Probability of captain hitting pirate's ship =

`P(A) = (1)/(2)`

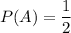
Probability of pirate hitting the captain's ship =

`P(B) = (1)/(6)`

Probability that both the pirate and the Captain hit each other's ships:

`P(A\cap B) = P(A)* P(B)`

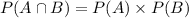
Putting values, we get,

`P(A\cap B) =(1)/(2)* (1)/(6) = (1)/(12) \\\\P(A\cap B) = 0 .083`

0.083 is the probability that both the pirate and the Captain hit each other's ships.