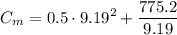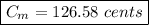Question

A rectangular bin is going to be made with a volume of 646 cm^3. The base of the bin will be a square and the top will be open. The cost of the material for the base is 0.5 cents per square centimeter, and the cost of the material for the sides is 0.3 cents per square centimeter. Determine the dimensions of the bin that will minimize the cost of manufacturing it. What is the minimum cost?

Answer 1

126.58 cents or $1.27

Explanation:

the math from above is correct they just want the answers in dollars

Answer 2

The base is a square of side 9.19 cm and the height is 7.66 cm

`C_m=126.58\ cents`

Explanation:

Optimization

We'll use simple techniques to find the optimum values that minimize the cost function given in the problem. Since the restriction is an equality, the derivative will come handy to find the critical points and then we'll prove they are a minimum.

First, we consider the shape of the rectangular bin has a square base and no top. Let x be the side of the base, thus the Area of the base is

`A_b=x^2`

Let y be the height of the box, thus each one of the four lateral sides of the box is a rectangle with sides x and y and the total lateral area is

`A_s=4xy`

The cost of the material used to manufacture the box is 0.5 cents per square centimeter of the base and 0.3 cents per square centimeter of the sides, thus the total cost to produce one box is

`C(x,y)=0.5x^2+0.3\cdot 4xy`

`C(x,y)=0.5x^2+1.2xy`

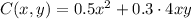
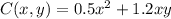
Note the cost is a two-variable function. We need to have it expressed as a single variable function. To achieve that, we use the volume provided as
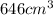`646 cm^3`. The volume of the box is the base times the height

`V=x^2y`

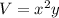
Using the value of the volume we have

`x^2y=646`

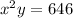
Solving for y

`\displaystyle y=(646)/(x^2)`

Replacing into the cost function, it only depends on one variable

`\displaystyle C(x)=0.5x^2+1.2x\cdot (646)/(x^2)`

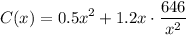
Operating

`\displaystyle C(x)=0.5x^2+ (775.2)/(x)`

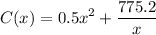
Taking the first derivative

`\displaystyle C'(x)=x-(775.2)/(x^2)`

Equating to 0

`\displaystyle x-(775.2)/(x^2)=0`

Solving

`\displaystyle x=\sqrt[3]{775.2}`

`x=9.19\ cm`

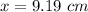
Now find the height

`\displaystyle y=(646)/(9.19^2)`

`y=7.66\ cm`

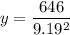
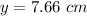
Find the second derivative

`\displaystyle C''(x)=1+(1550.4)/(x^3)`

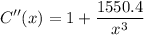
Since this value is positive, for all x positive, the function has a minimum at the critical point.

Thus, the minimum cost is

`\displaystyle C_m=0.5\cdot 9.19^2+ (775.2)/(9.19)`

`\boxed{C_m=126.58\ cents}`