Answer:
Part (A)
- 1. Maximum revenue: $450,000
Part (B)
- 2. Maximum protit: $192,500
- 3. Production level: 2,300 television sets
- 4. Price: $185 per television set
Part (C)
- 5. Number of sets: 2,260 television sets.
- 6. Maximum profit: $183,800
- 7. Price: $187 per television set.
Step-by-step explanation:
0. Write the monthly cost and price-demand equations correctly:
Cost:
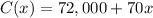
Price-demand:
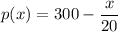
Domain:

1. Part (A) Find the maximum revenue
Revenue = price × quantity
Revenue = R(x)
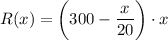
Simplify
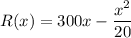
A local maximum (or minimum) is reached when the first derivative, R'(x), equals 0.
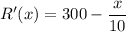
Solve for R'(x)=0
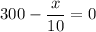
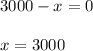
Is this a maximum or a minimum? Since the coefficient of the quadratic term of R(x) is negative, it is a parabola that opens downward, meaning that its vertex is a maximum.
Hence, the maximum revenue is obtained when the production level is 3,000 units.
And it is calculated by subsituting x = 3,000 in the equation for R(x):
- R(3,000) = 300(3,000) - (3000)² / 20 = $450,000
Hence, the maximum revenue is $450,000
2. Part (B) Find the maximum profit, the production level that will realize the maximum profit, and the price the company should charge for each television set.
i) Profit(x) = Revenue(x) - Cost(x)
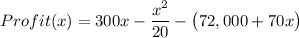
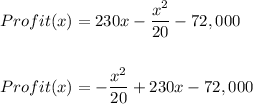
ii) Find the first derivative and equal to 0 (it will be a maximum because the quadratic function is a parabola that opens downward)
- Profit' (x) = -x/10 + 230
Thus, the production level that will realize the maximum profit is 2,300 units.
iii) Find the maximum profit.
You must substitute x = 2,300 into the equation for the profit:
- Profit(2,300) = - (2,300)²/20 + 230(2,300) - 72,000 = 192,500
Hence, the maximum profit is $192,500
iv) Find the price the company should charge for each television set:
Use the price-demand equation:
- p(2,300) = 300 - 2,300 / 20
Therefore, the company should charge a price os $185 for every television set.
3. Part (C) If the government decides to tax the company $4 for each set it produces, how many sets should the company manufacture each month to maximize its profit? What is the maximum profit? What should the company charge for each set?
i) Now you must subtract the $4 tax for each television set, this is 4x from the profit equation.
The new profit equation will be:
- Profit(x) = -x² / 20 + 230x - 4x - 72,000
- Profit(x) = -x² / 20 + 226x - 72,000
ii) Find the first derivative and make it equal to 0:
- Profit'(x) = -x/10 + 226 = 0
Then, the new maximum profit is reached when the production level is 2,260 units.
iii) Find the maximum profit by substituting x = 2,260 into the profit equation:
- Profit (2,260) = -(2,260)² / 20 + 226(2,260) - 72,000
Hence, the maximum profit, if the government decides to tax the company $4 for each set it produces would be $183,800
iv) Find the price the company should charge for each set.
Substitute the number of units, 2,260, into the equation for the price:
- p(2,260) = 300 - 2,260/20
That is, the company should charge $187 per television set.