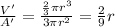Answer:
Volume:
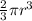
Ratio:
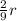
Explanation:
First of all, we need to find the volume of the hemispherical tank.
The volume of a sphere is given by:
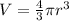
where
r is the radius of the sphere
V is the volume
Here, we have a hemispherical tank: a hemisphere is exactly a sphere cut in a half, so its volume is half that of the sphere:

Now we want to find the ratio between the volume of the hemisphere and its surface area.
The surface area of a sphere is

For a hemisphere, the area of the curved part of the surface is therefore half of this value, so
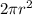 . Moreover, we have to add the surface of the base, which is
. Moreover, we have to add the surface of the base, which is
 . So the total surface area of the hemispherical tank is
. So the total surface area of the hemispherical tank is
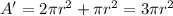
Therefore, the ratio betwen the volume and the surface area of the hemisphere is