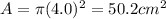Question

What is the largest area of a circle that can be made from a piece of thread 25.2cm long assume no knot

Answer 1

`50.2 cm^2`

Step-by-step explanation:

Here we want to find the largest area of a circle that can be made from a piece of thread 25.2 cm long, assume no knot .

This means that the circumference of the circle must equal to the length of the thread, so we can write:

L = 25.2 cm

And the circumference can be written as:

`2\pi r = L = 25.2`

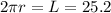
where

r is the radius of the circle

Solving for r, we find the radius:

`r=(L)/(2\pi)=(25.2)/(2\pi)=4.0 cm`

The area of a circle is given by the formula

`A=\pi r^2`

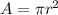
So in this case, we find:

`A=\pi (4.0)^2=50.2 cm^2`