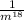Question

Simplify the expression.

(m^-1 m^5/m^-2)^-3

a)- 3m^4/m^2
b)-m^216
c)m^18
d)1/m^18

Answer 1

d) 1/m^18

Step-by-step explanation:
(m^-2/m-1m5)^3
m^-6/m^-3m^15
1/m^6 / m^15/m^3
1/m^6 / m^12
1/m^6 (1/m^12)
1/m^18

let me know if you want clarification

Answer 2

`D)(1)/(m^(18) )`

Step-by-step explanation:

Multiply
`m^(-1)` by
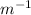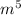`m^(5)` by adding the exponents.

Use the power rule
`a^(m) a^(n) =a^(m+n)` to combine exponents.

`((m^(-1+5) )/(m^(-2) ) )^(-3) \\`

Add -1 and 5.

`((m^(4) )/(m^(-2) ) )^(-3)`

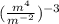
Move
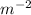`m^(-2)` to the numerator using the negative exponent rule
`(1)/(b^(-n) ) =b^(n)`.

`(m^(4) m^(2) )`

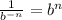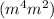
Multiply
`m^(4)` by
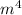`m^(2)` by adding the exponents.

Use the power rule
`a^(m) a^(n) =a^(m+n)` to combine exponents.

`(m^(4+2) )^(-3)`

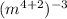
Add 4 and 2.

`(m^(6) )^(-3)`

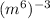
Multiply the exponents in
`(m^6)^(-3)`

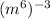
Apply the rule and multiply exponents,
`(a^m)^n=a^(mn)`.

`m^(6*-3)`

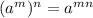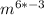
Multiply 6 by -3.

`m^(-18)`

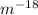
Rewrite the expression using the negative exponent rule
`b^(-n) =(1)/(b^(n) )`.

`(1)/(m^(18) )`