Question

The graph of f(x) = -x2 - 2x + 8 is shown. Which of the following describes all solutions for f(x)?

O(x -x2 - 2x + 8) for all real numbers
O(-4,0), (-1,9), (0, 8), (2.0)
O(-4.0), (2,0)
O(x, y) for all real numbers

Answer 1

`f(x) = -x^2 - 2x + 8`

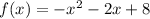
y-intercept is when x = 0:

`\implies f(0) = -(0)^2 - 2(0) + 8=8`

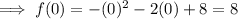
So the y-intercept is (0, 8)

x-intercepts are when f(x) = 0:

`\implies f(x)=0`

`\implies -x^2 - 2x + 8=0`

`\implies x^2 + 2x - 8=0`

`\implies x^2 - 2x + 4x - 8=0`

`\implies x(x - 2) + 4(x-2)=0`

`\implies (x+4)(x - 2)=0`

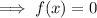
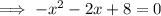
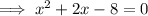
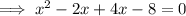
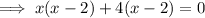
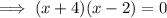
Therefore:

`\implies (x+4)=0 \implies x=-4`

`\implies (x-2)=0 \implies x=2`

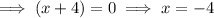
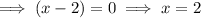
So the x-intercepts are (-4, 0) and (2, 0)

The vertex is the turning point of the parabola. The x-value of the vertex is the x-value between the 2 zeros (x-intercepts).

Therefore, the x-value of the vertex is
`\sf (x_1+x_2)/(2)=(-4+2)/(2)=-1`

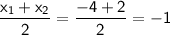
Substituting x = -1 into the function:

`\implies f(-1) = -(-1)^2 - 2(-1) + 8=9`

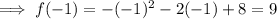
So the vertex is (-1, 9)

The domain is the input values, so x = all real numbers.

The range is the output values, so the range is
`f(x)\leq 9`

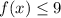
Therefore, (-4, 0), (-1, 9), (0, 8) and (2, 0) are solutions of the function, HOWEVER they are not ALL the solutions.

All solutions of the function are:

`(x, -x^2 - 2x + 8)` for all real numbers of x