Question

Two light waves are initially in phase and have the same wavelength, 470 nm. They enter two different media of identical lengths of 2.50 µm. If n1 = 1.2 and n2 = 1.5, what is the effective phase difference of the waves when they exit the media? Group of answer choices 3.7 rad 10 rad 1.6 rad 0.6 rad 0 rad

Answer 1

= 3.7 radians = effective phase difference

Step-by-step explanation:

Wavelength given =
`\lambda= 470 nm`

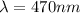
n1 = 1.2 and n2 = 1.5

The light rays are traveling at different speeds inside the 2 different layers.

So effective optical path is given by n d

where n is the refractive index of the medium and

d is the length traveled by the light ray in the medium.

The path difference introduced because of 2 different medium is

length = distance traveled by the wave = d = 2.5 x 10^-6 m

Phase difference =
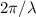`2 \pi / \lambda` * path difference

path difference = (n₂ - n₁ ) d

= (1.5 - 1.2) (2.5 x 10⁻⁶)

= 7.5 x 10⁻⁷ m

phase difference = 2 pi / (470 x 10⁻⁹) * (7.5 x 10⁻⁷) = 10 rad

2 pi radians is 360 degrees

10 radians = 573 degrees

Phase difference = 573 - 360 = 212 degrees

= 3.7 radians = effective phase difference