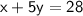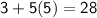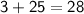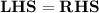Question

HELP ME PLEASE!!

Solve the simultaneous equations.
x + 2y = 13
x + 5y = 28

x =
y= ​

Answer 1

• x+2y=13--(1)
• x+5y=28--(2)

Let's use elimination method

• Subtract eq(2) from eq(1)

We get

`\\ \rm\Rrightarrow 2y-5y=13-28`

`\\ \rm\Rrightarrow -3y=-15`

`\\ \rm\Rrightarrow y=(-15)/(-3)`

`\\ \rm\Rrightarrow y=5`

Putting on eq(1)

`\\ \rm\Rrightarrow x+2y=13`

`\\ \rm\Rrightarrow x+2(5)=13`

`\\ \rm\Rrightarrow x+10=13`

`\\ \rm\Rrightarrow x=13-10`

`\\ \rm\Rrightarrow x=3`

• (x,y)=(3,5)

`\LARGE{\underbrace{\underline{\rm{Verification:-}}}}`

`\\ \rm\Rrightarrow x+2y=13`

`\\ \rm\Rrightarrow 3+2(5)=13`

`\\ \rm\Rrightarrow 3+10=13`

`\\ \rm\Rrightarrow 13=13`

And

`\\ \rm\Rrightarrow x+5y=28`

`\\ \rm\Rrightarrow 3+5(5)=28`

`\\ \rm\Rrightarrow 3+25=28`

`\\ \rm\Rrightarrow 28=28`

Hence verified!

Answer 2

`\bold{\huge{\underline{ Solution }}}`

Given :-

• Here, We have given two equations that is ,
• 
  `\sf{ x + 2y = 13\:\: and\:\: x + 5y = 28}`

To Find :-

• We have to find the value of x and y.

Concept used :-

• Linear equations are those equations which having highest power of degree as 1 .
• Linear equations can be solved by subsitute method, elimination method and cross multiplication method.
• Here, I have used substitution method to make calculation easier.
• In subsitute method, you have to subsitute the value of one variable of eq(1) in eq(2) .

Let's Begin :-

Here,

• We have two equations, let consider these two equations as eq(1) and eq(2) that is,

`\sf{ x + 2y = 13 ...eq(1)}`

`\sf{ x + 5y = 28 ...eq(2)}`

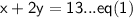
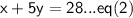
By solving eq(1) :-

`\sf{ x + 2y = 13 }`

`\sf{ x = 13 - 2y ...eq(3)}`

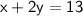
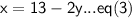
Subsitute eq(3) in eq(2) :-

`\sf{ (13 - 2y) + 5y = 28 }`

`\sf{ 13 - 2y + 5y = 28 }`

`\sf{ 13 + 3y = 28 }`

`\sf{ 3y = 28 - 13 }`

`\sf{ 3y = 28 - 13 }`

`\sf{ 3y = 15 }`

`\sf{ y = }{\sf{( 15)/(3)}}`

`\sf{ y = }{\sf{\cancel{( 15)/(3)}}}`

`\bold{ y = 5 }`

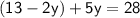
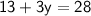
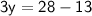
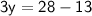
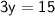
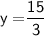
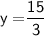
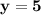
Thus, The value of y is 5

Now,

Subsitute the value of y in eq(1) :-

`\sf{ x + 2(5) = 13 }`

`\sf{ x + 10 = 13 }`

`\sf{ x = 13 - 10 }`

`\bold{ x = 3 }`

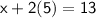
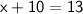
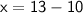
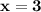
Hence, The value of x and y are 5 and 3 .

`\bold{\huge{\underline{ Let's \: Verify }}}`

Equation 1 :-

`\sf{ x + 2y = 13 }`

`\sf{ 3 + 2(5) = 13 }`

`\sf{ 3 + 10 = 13 }`

`\sf{ 13 = 13 }`

`\bold{ LHS = RHS }`

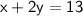
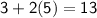
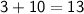
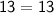
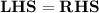
Equation 2 :-

`\sf{ x + 5y = 28 }`

`\sf{ 3 + 5(5) = 28 }`

`\sf{ 3 + 25 = 28 }`

`\sf{ 28 = 28 }`

`\bold{ LHS = RHS }`