A function that represents the exponential function f(x)= 3 to the power of x after a vertical stretch by a factor of 8 and a reflection across the x-axis is
 .
.
Explanation:
Here we have to make A function that represents the exponential function f(x)= 3 to the power of x after a vertical stretch by a factor of 8 and a reflection across the x-axis . Let's find out:
We have ,
- A function that represents the exponential function f(x)= 3 to the power of x
Following is the equation for above statement :
⇒
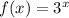
- A vertical stretch by a factor of 8
Following is the equation for above statement :
⇒
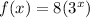
- A reflection across the x-axis
Following is the equation for above statement :
⇒
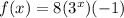
⇒

Therefore , A function that represents the exponential function f(x)= 3 to the power of x after a vertical stretch by a factor of 8 and a reflection across the x-axis is
 .
.