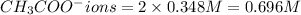Question

In the laboratory you dissolve 14.8 g of chromium(II) acetate in a volumetric flask and add water to a total volume of 250 mL. What is the molarity of the solution? M. What is the concentration of the chromium(II) cation? M. What is the concentration of the acetate anion? M. Submit AnswerRetry Entire Group

Answer 1

Answer: a) The molarity of the solution is 0.348 M

b) The concentration of the chromium(II) cation is 0.348 M

c) The concentration of the acetate anion is 0.696 M

Step-by-step explanation:

Molarity of a solution is defined as the number of moles of solute dissolved per Liter of the solution.

`Molarity=(n* 1000)/(V_s)`

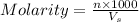
where,

n = moles of solute

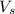`V_s` = volume of solution in ml = 150 ml

moles of solute =
`\frac{\text {given mass}}{\text {molar mass}}=(14.8g)/(170g/mol)=0.0870moles`

Now put all the given values in the formula of molality, we get

`Molality=(0.0870* 1000)/(250)=0.348M`

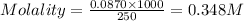
Thus molarity of solution is 0.348 M

`Cr(CH_3COO)_2\rightarrow Cr^(2+)+2CH_3COO^-`

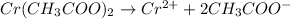
According to stoihiometry;

1 mole of chromium acetate gives 1 mole of chromium ions

Thus molarity of
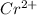`Cr^(2+)` ions = 0.348 M

1 mole of chromium acetate gives 2 moles of acetate ions

Thus molarity of
`CH_3COO^- ions =2* 0.348 M=0.696M`