Answer:
(a).
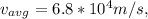 is 0.02% the speed of light.
is 0.02% the speed of light.
(b).
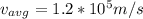 , is 0.04% the speed of light.
, is 0.04% the speed of light.
(c).
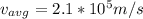 is 0.07% the speed of light.
is 0.07% the speed of light.
Step-by-step explanation:
The average kinetic energy is related to the thermal energy of the electrons in a conductor by the the relation
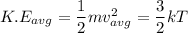 ,
,
where
 is the mass of the electrons,
is the mass of the electrons,
 is their average velocity,
is their average velocity,
 is the temperature of the conductor, and
is the temperature of the conductor, and
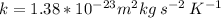 is the Boltzmann constant.
is the Boltzmann constant.
The equation, when solved for
 , gives
, gives
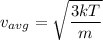
(a),
For
 , the thermal (average) velocity
, the thermal (average) velocity
 is
is


which when compared to the speed of light is
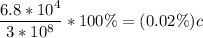
0.02% the speed of light.
(b).
Similarly, for
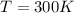


which is
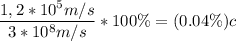
0.04% the speed of light.
(c).
Finally, for
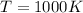

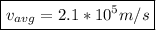
which is

0.07% the speed of light.
We see that the average electron velocities we obtain are always less than 1% the speed of light, which means relativistic effects are negligible, for they are apparent at about 25% the speed of light.