Question

Jerry and Meena are riding their bicycles through the city to meet at the park, as shown on the coordinate plane. On the coordinate plane, north is in the positive y-direction, and 1 unit represents 1 city block. Jerry starts at the point (2, −5) and rides north toward the park at the point (2, 1). Meena starts at east of the park at the point (5, 1) and rides west toward the park. How far does each person travel to reach the park?

Answer 1

Part 1) Jerry travel 6 city blocks to reach the park

Part 2) Meena travel 3 city blocks to reach the park

Explanation:

The complete question in the attached figure

we know that

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

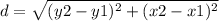
step 1

Find the distance that Jerry travel to reach the park

we have the ordered pairs

(2,-5) and (2,1)

substitute in the formula

`d=\sqrt{(1+5)^(2)+(2-2)^(2)}`

`d=\sqrt{(6)^(2)+(0)^(2)}`

`d=6\ units`

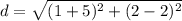
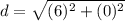
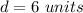
Convert to city blocks (1 unit represents 1 city block)

so

`d=6\ city\ blocks`

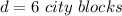
therefore

Jerry travel 6 city blocks to reach the park

step 2

Find the distance that Meena travel to reach the park

we have the ordered pairs

(2,1) and (5,1)

substitute in the formula

`d=\sqrt{(1-1)^(2)+(5-2)^(2)}`

`d=\sqrt{(0)^(2)+(3)^(2)}`

`d=3\ units`

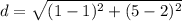
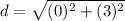
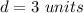
Convert to city blocks (1 unit represents 1 city block)

so

`d=3\ city\ blocks`

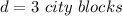
therefore

Meena travel 3 city blocks to reach the park