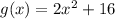The equation of g(x) is
Step-by-step explanation:
Given that the function f(x) is
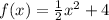
Also, given that the function g(x) is a vertical stretch of f(x) by a factor of 4.
We need to determine the equation of g(x)
Equation of g(x):
The vertical stretch of the function can be determined by multiplying the factor 4 with the function f(x).
Thus, we have,
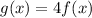
Substituting the values,we have,
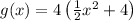
Simplifying the values, we get,
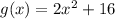
Hence, the equation of g(x) is