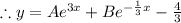Question

Write the given differential equation in the form L(y) = g(x), where L is a linear differential operator with constant coefficients. If possible, factor L. (Used for the differential operator.) 3y'' − 8y' − 3y = 4

Answer 1

The complete solution is

`\therefore y= Ae^(3x)+Be^(-\frac13 x)-\frac43`

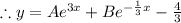
Explanation:

Given differential equation is

3y"- 8y' - 3y =4

The trial solution is

`y = e^(mx)`

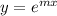
Differentiating with respect to x

`y'= me^(mx)`

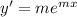
Again differentiating with respect to x

`y''= m ^2 e^(mx)`

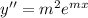
Putting the value of y, y' and y'' in left side of the differential equation

`3m^2e^(mx)-8m e^(mx)- 3e^(mx)=0`

`\Rightarrow 3m^2-8m-3=0`

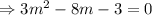
The auxiliary equation is

`3m^2-8m-3=0`

`\Rightarrow 3m^2 -9m+m-3m=0`

`\Rightarrow 3m(m-3)+1(m-3)=0`

`\Rightarrow (3m+1)(m-3)=0`

`\Rightarrow m = 3, -\frac13`

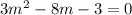
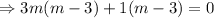
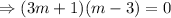
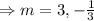
The complementary function is

`y= Ae^(3x)+Be^(-\frac13 x)`

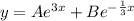
y''= D², y' = D

The given differential equation is

(3D²-8D-3D)y =4

⇒(3D+1)(D-3)y =4

Since the linear operation is

L(D) ≡ (3D+1)(D-3)

For particular integral

`y_p=\frac 1{(3D+1)(D-3)} .4`

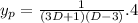
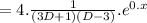`=4.\frac 1{(3D+1)(D-3)} .e^(0.x)` [since
`e^(0.x)=1`]

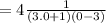`=4(1)/((3.0+1)(0-3))` [ replace D by 0 , since L(0)≠0]

`=-\frac43`

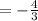
The complete solution is

y= C.F+P.I

`\therefore y= Ae^(3x)+Be^(-\frac13 x)-\frac43`