Answer:
Rowing rate of the guide in calm water is 6 mph.
Explanation:
Let the rowing rate of the guide is x mph in the calm water.
Rate of river's current = 4 mph
Therefore, speed of the boat upstream = (x - 4) mph
and speed of the river downstream = (x + 4) mph
Time taken to row 5 miles upstream =
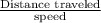
=
 hours
hours
Time taken to row 5 miles downstream =
 hours
hours
Since total time spent to row down and come back is = 3 hours
So
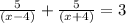
![5[(x+4+x-4)/((x-4)(x+4))]=3](https://img.qammunity.org/2021/formulas/mathematics/high-school/t0or6q2nmo3tpdhqa30osfp7td041nsa47.png)
5(2x) = 3(x - 4)(x + 4)
10x = 3(x² - 16)
3x² - 10x - 48 = 0
From quadratic formula,
x =
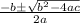
From our equation,
a = 3, b = -10 and c = -48
Now we plug in these values in the formula,
x =

=
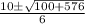
=
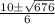
=
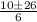
= 6, -2.67 mph
Since speed can not be negative so x = 6 mph will be the answer.
Therefore, rowing rate of the guide in calm water is 6 mph.