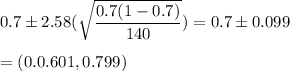Answer:
a) 0.7
b) 0.075
c) (0.0.601,0.799)
Explanation:
We are given the following in the question:
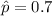
Sample size, n = 140
(a) Point estimate
The best point estimate for population proportion is the sample proportion.
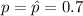
(b) Margin of error
Formula:
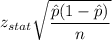
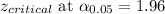
Putting values, we get,
Margin of error =
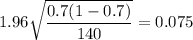
(c) 99% confidence interval
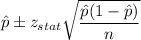
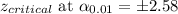
Putting the values, we get: