Answer:
a < -

Explanation:
Using the discriminant Δ = b² - 4ac
For a polynomial to have 2 real roots
b² - 4ac > 0
Given
p(x) = (a + 1)x² + 2ax + (a + 2)
with a = a + 1, b = 2a and c = a + 2, then
(2a)² - 4(a + 1)(a + 2) > 0 ← expand and simplify left side
4a² - 4(a² + 3a + 2) > 0
4a² - 4a² - 12a - 8 > 0
- 12a - 8 > 0 ( add 8 to both sides )
- 12a > 8
Divide both sides by - 12, reversing the symbol as a result of dividing by a negative quantity
a <
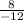 , that is
, that is
a < -
