Question

The local supermarket buys lettuce each day to ensure really fresh produce. Each morning any lettuce that is left from the previous day is sold to a dealer that resells it to farmers who use it to feed their animals. This week the supermarket can buy fresh lettuce for $8.00 a box. The lettuce is sold for $16.00 a box and the dealer that sells old lettuce is willing to pay $1.80 a box. Past history says that tomorrow's demand for lettuce averages 245 boxes with a standard deviation of 38 boxes. How many boxes of lettuce should the supermarket purchase tomorrow

Answer 1

The supermarket should purchase 251 boxes of lettuce tomorrow.

Explanation:

Let X = number of boxes of lettuce that the supermarket should purchase tomorrow

The information provided is:

Cost price per box = $8.00

Selling price per box = $16.00

The amount paid by the dealer for selling old lettuce per box (Salvage price) = $1.80

The mean number of boxes demanded for lettuce is, μ = 245.

The standard deviation of the number of boxes demanded for lettuce is, σ = 38.

Excess cost per box is,

`C_(E)=Cost\ price-Salvage\ price=8.00-1.80=\$6.20`

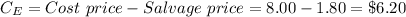
Shortage cost per box is,

`C_(S)=Selling\ price-Cost\ price=16.00-8.00=\$8.00`

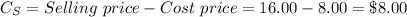
Compute the level of service as follows:

`Level=(C_(S))/(C_(S)+C_(E))=(8)/(8+6.2)=0.5634`

For the service level value the z-value is,

z = 0.1596

*Use a z-table.

Compute the value of x as follows:

`z=(x-\mu)/(\sigma)\\0.1596=(x-245)/(38)\\x=245+(0.1596*38)\\x=251.0648\\\approx251`

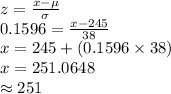
Thus, the supermarket should purchase 251 boxes of lettuce tomorrow.