Question

The diagram shows a sector of a circle of radius 6 units, formed from an angle of size 305°.

Find the exact length of the arc.
3050

Answer 1

The length of the arc is 31.92 units

Step-by-step explanation:

Given that the sector of a circle of radius 6 units.

The angle is given by
`\theta=305^(\circ)`

Arc length of the circle:

The arc length of the circle can be determined using the formula,

`\ {arc\ length}=2 \pi r\left((\theta)/(360)\right)`

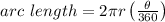
where r = 6 and
`\theta=305^(\circ)`

Substituting the values, we have,

`\ {arc\ length}=2 \pi (6)\left((305)/(360)\right)`

Multiplying the numerator, we have,

`\ {arc\ length}=(3660 \pi)/(360)`

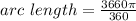
Substituting
`\pi=3.14`, we have,

`\ {arc\ length}=(3660(3.14))/(360)`

Multiplying the terms, we get,

`\ {arc\ length}=(11492.4)/(360)`

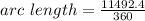
Dividing, we get,

`\ {arc\ length}=31.92 \ units`

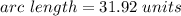
Thus, the arc length of the circle is 31.92 units