Answer:
P(0.16 <
 < 0.24) = 0.9546 or 95.5%
< 0.24) = 0.9546 or 95.5%
Explanation:
We are given that 20% of the residents in a certain state support an increase in the property tax. An opinion poll will randomly sample 400 state residents and will then compute the proportion in the sample that support a property tax increase.
Let p = % of the residents in a certain state that support an increase in the property tax = 20%
 = % of the residents in a certain state that support an increase in the
= % of the residents in a certain state that support an increase in the
property tax in a sample of 400 residents
The one-sample z sore probability distribution for sample proportion is given by;
Z =
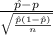 ~ N(0,1) where, n = sample residents = 400
~ N(0,1) where, n = sample residents = 400
So, probability that sample proportion will lie between 0.16 and 0.24 is given by = P(0.16 <
 < 0.24)
< 0.24)
P(0.16 <
 < 0.24) = P(
< 0.24) = P(
 < 0.24) - P(
< 0.24) - P(
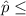 0.16)
0.16)
P(
 < 0.24) = P(
< 0.24) = P(
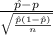 <
<
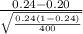 ) = P(Z < 1.87) = 0.96926
) = P(Z < 1.87) = 0.96926
P(

 0.16) = P(
0.16) = P(
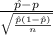

 ) = P(Z < -2.18) = 1 - P(Z
) = P(Z < -2.18) = 1 - P(Z
 2.18)
2.18)
= 1 - 0.98537 = 0.01463
Therefore, P(0.16 <
 < 0.24) = 0.96926 - 0.01463 = 0.9546
< 0.24) = 0.96926 - 0.01463 = 0.9546
Hence, it is 95.5% likely that the sample proportion will lie between 0.16 and 0.24.