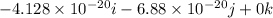Answer:
The force that the wire exerts on the electron is
Step-by-step explanation:
Given that,
Current = 8.60 A
Velocity of electron
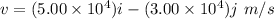
Position of electron = (0,0.200,0)
We need to calculate the magnetic field
Using formula of magnetic field
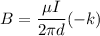
Put the value into the formula
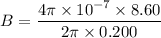


We need to calculate the force that the wire exerts on the electron
Using formula of force
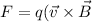
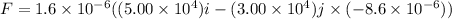
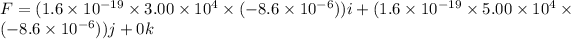

Hence, The force that the wire exerts on the electron is