Question

Given the lengths of two sides of a triangle, find the range for the length of the third side (between what two numbers should the length of the third side be). Write the inequalities for each case. a 8 and 13

Answer 1

Length of third side of the triangle should be between 6 and 20.

Explanation:

Case 1:

Given:

First side of the triangle = 8

Second side of the triangle = 13

we need to find the range of the third side of the triangle.

Solution:

Now we know that;

By Triangle inequality theorem.

"The length of the third side should be greater than difference of other two sides and less than sum of other two sides."

by using this property we get;

Length of the third side <
`13+8`

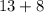
Length of the third side < 21

Length of the third side >
`13-8`

Length of the third side > 5

Hence Length of third side of the triangle should be between 6 and 20.

Since there is only case given we had solve for the same for rest of the cases use the same property and find the length of the third side of the triangle.