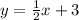Question

(05.02)

Look at the graph shown:
lo
2
3
4
5
Which equation best represents the line?
Oy = 3x + 3
Oy = x-3
Oy = kx +3
Oy = 3x + 3

Answer 1

C

Explanation:

Answer 2

`y=(1)/(2)x+3`

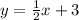
Explanation:

Let the equation of the line be:
`y=mx+b`

where m is the slope and b is the y-intercept.

The given straight line has a y-intercept at
`b=3`

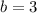
The equation is of the form
`y=mx+3`

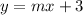
The line passes through the point (2,4).

Let us substitute x=2, and y=4 to find the value of m.

`4=m(2)+3`

`4=2m+3\\4-3=2m\\1=2m\\m=(1)/(2)`

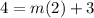
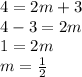
The slope is
`m=(1)/(2)`

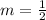
Hence the equation of the line is
`y=(1)/(2)x+3`