Question

Suppose we have a set of paired data and let d = x_after - x_before. The null hypothesis is H_o :μd = 0, the alternative hypothesis is H_a : μd =/ 0 nd the population standard deviation sigma is not known. Jordan collects a sample of size n=9 and computes d = 2.2 and Sd=3.3.a) What is the value of the test statistic?b) The probability of obtaining a test statistic greater than Jordan's test statistic is 0.0403. Use this probability to determine the P-value for Jordan's hypothesis test. (Round to three decimal places.)

Answer 1

(a) The value of the test statistic is 2.

(b) The p-value of the test is, 0.081.

Explanation:

The dependent t-test (also known as the paired t-test or paired-samples t-test) compares the two means of associated groups to conclude if there is a statistically significant difference amid these two means.

We use the paired t-test if we have two measurements on the same item, person or thing.

The hypothesis for the test is defined as:

H₀:
`\mu_(d)=0` vs. Hₐ:
`\mu_(d)\\eq 0`.

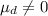(a)

The t-statistic is given by:

`t=(d)/(SD/√(n))`

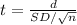
Given:

d = 2.2

SD = 3.3

n = 9

Compute the value of t as follows:

`t=(d)/(SD/√(n))=(2.2)/(3.3/√(9))=2`

Thus, the value of the test statistic is 2.

(b)

The level of significance is, α = 0.0403.

Compute the p value of the test statistic as follows:

The test statistic value is, t = 2

The degrees of freedom is, (n - 1) = 9 - 1 = 8.

Use a t-table for the p-value.

`p-value=0.081`

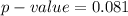
Conclusion:

As the p-value = 0.081 > α = 0.0403, the null hypothesis was failed to be rejected.