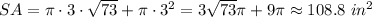Question

Find the Surface area of each figure to the nearest tenth.

Answer 1

a. 800 square inches

b. 108.8 square inches

Explanation:

a. The diagram shows the square pyramid with the square side length of 16 inches, the height of 15 inches and slant height of 17 inches.

The surface area of the square pyramid is

`SA=4S_(triangle)+S_(base)`

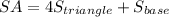
Find the area of the base and of each lateral triangle:

`S_(base)=16* 16=256\ in^2\\ \\S_(triangle)=(1)/(2)* 16* 17=136\ in^2`

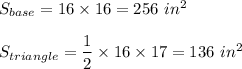
Therefore,

`SA=4\cdot 136+256=800\ in^2`

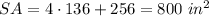
b. The diagram shows the cone with the base radius of 3 inches and height of 8 inches.

The surface area of the cone is

`SA=\pi Rl+\pi R^2,`

where R is the base radius and l is the slant height.

First, find the slant height using the Pythagorean theorem:

`l^2=H^2+R^2\\ \\l^2=3^2+8^2\\ \\l^2=9+64\\ \\l^2=73\\ \\l=√(73)\ in`

Then, the surface area is

`SA=\pi \cdot 3\cdot √(73)+\pi \cdot 3^2=3√(73)\pi +9\pi \approx 108.8\ in^2`