So , Inverse of
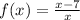 is
is
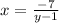 .
.
Explanation:
An inverse function is a function that "reverses" another function: if the function f applied to an input x gives a result of y, then applying its inverse function g to y gives the result x, and vice versa, i.e., f(x) = y if and only if g(y) = x. As an example, consider the real-valued function of a real variable given by f(x) = 5x − 7. In functional notation this inverse function would be given by,
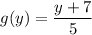
With y = 5x − 7 we have that f(x) = y and g(y) = x. Here we have
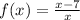
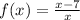
⇒
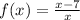
⇒
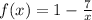
Let f(x) = y:
⇒
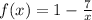
⇒
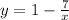
⇒
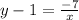
⇒
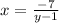
So , Inverse of
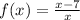 is
is
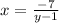 .
.