Question

The equation of function h is h... PLEASE HELP MATH

Answer 1

Part A: the value of h(4) - m(16) is -4

Part B: The y-intercepts are 4 units apart

Part C: m(x) can not exceed h(x) for any value of x

Explanation:

Let us use the table to find the function m(x)

There is a constant difference between each two consecutive values of x and also in y, then the table represents a linear function

The form of the linear function is m(x) = a x + b, where

• a is the slope of the function
• b is the y-intercept

The slope = Δm(x)/Δx

∵ At x = 8, m(x) = 2

∵ At x = 10, m(x) = 3

∴ The slope =
`(3-2)/(10-8)=(1)/(2)`

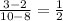
∴ a =
`(1)/(2)`

- Substitute it in the form of the function

∴ m(x) =
`(1)/(2)` x + b

- To find b substitute x and m(x) in the function by (8 , 2)

∵ 2 =
`(1)/(2)` (8) + b

∴ 2 = 4 + b

- Subtract 4 from both sides

∴ -2 = b

∴ m(x) =
`(1)/(2)` x - 2

Now let us answer the questions

Part A:

∵ h(x) =
`(1)/(2)` (x - 2)²

∴ h(4) =
`(1)/(2)` (4 - 2)²

∴ h(4) =
`(1)/(2)` (2)²

∴ h(4) =
`(1)/(2)`(4)

∴ h(4) = 2

∵ m(x) =
`(1)/(2)` x - 2

∴ m(16) =
`(1)/(2)` (16) - 2

∴ m(16) = 8 - 2

∴ m(16) = 6

- Find now h(4) - m(16)

∵ h(4) - m(16) = 2 - 6

∴ h(4) - m(16) = -4

Part B:

The y-intercept is the value of h(x) at x = 0

∵ h(x) =
`(1)/(2)` (x - 2)²

∵ x = 0

∴ h(0) =
`(1)/(2)` (0 - 2)²

∴ h(0) =
`(1)/(2)` (-2)² =

∴ h(0) = 2

∴ The y-intercept of h(x) is 2

∵ m(x) =
`(1)/(2)` x - 2

∵ x = 0

∴ m(0) =
`(1)/(2)` (0) - 2 = 0 - 2

∴ m(0) = -2

∴ The y-intercept of m(x) is -2

- Find the distance between y = 2 and y = -2

∴ The difference between the y-intercepts of the graphs = 2 - (-2)

∴ The difference between the y-intercepts of the graphs = 4

∴ The y-intercepts are 4 units apart

Part C:

The minimum/maximum point of a quadratic function f(x) = a(x - h) + k is point (h , k)

Compare this form with the form of h(x)

∵ h = 2 and k = 0

∴ The minimum point of the graph of h(x) is (2 , 0)

∵ k is the minimum value of f(x)

∴ 0 is the minimum value of h(x)

∴ The domain of h(x) is all real numbers

∴ The range of h(x) is h(x) ≥ 2

∵ m(8) = 2

∵ m(14) = 5

∵ h(8) =
`(1)/(2)` (8 - 2)² = 18

∵ h(14) =
`(1)/(2)` (14 - 2)² = 72

∴ h(x) is always > m(x)

∴ m(x) can not exceed h(x) for any value of x

Look to the attached graph for more understand

The blue graph represents h(x)

The green graph represents m(x)

The blue graph is above the green graph for all values of x, then there is no value of x make m(x) exceeds h(x)