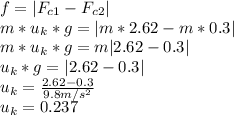Question

If a car takes a banked curve at less than the ideal speed, friction is needed to keep it from sliding toward the inside of the curve (a problem on icy mountain roads). (a) Calculate the ideal speed to take a 100.0 m radius curve banked at . (b) What is the minimum coefficient of 15.0°friction needed for a frightened driver to take the same curve at 20.0 km / h?

Answer 1

`(a) v=16.2m/s\\(b) u_(k)=0.237`

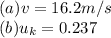
Step-by-step explanation:

Given data

`r=100.0m\\\alpha =15.0^(o)\\v_(2)=20.0km/h=5.6m/s`

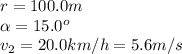
For Part (a) Speed

The speed v is given by

`tan(\alpha )=(v^2)/(r.g)\\ v^2=tan(\alpha )*r.g\\v=√(tan(\alpha )*r.g)\\ v=√(tan(15)*100.0m*9.81m/s^2)\\ v=16.2m/s`

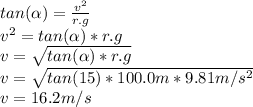
For Part (b) minimum coefficient of friction

To determine the friction of coefficient we know that friction force f is given by:

`f=u_(k).N=u_(k).m.g`

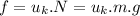
The first centripetal force Fc₁ is given by:

`F_(c1)=ma_(c1)\\F_(c1)=(m.v_(1)^2)/(r)\\ F_(c1)=(m.(16.2m/s)^2)/(100m)\\ F_(c1)=m.2.62`

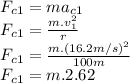
The second centripetal force Fc₂ is given by:

`F_(c2)=ma_(c2)\\F_(c2)=(m.v_(2)^2)/(r)\\ F_(c2)=(m.(5.6m/s)^2)/(100m)\\ F_(c2)=m.0.3`

The additional friction force is given by:

`f=|F_(c1)-F_(c2)|\\m*u_(k)*g=|m*2.62-m*0.3|\\m*u_(k)*g=m|2.62-0.3|\\u_(k)*g=|2.62-0.3|\\u_(k)=(2.62-0.3)/(9.8m/s^2)\\u_(k)=0.237`